Decimal Numbers. Real Numbers. Scientific notation
Real Numbers
As we said before, there are numbers that we can’t express as the quotient of other two numbers, such as:
π = 3.1415.., e = 2.7182.. , √2 = 1.4142.., golden ratio = φ =(1+v5)/2 = 1.61803..
These numbers are called Irrational Numbers, and if we add them to the Rational Numbers we obtain the Real Numbers, R , which we can represent in a straight line.

Now, we have these sets of numbers:
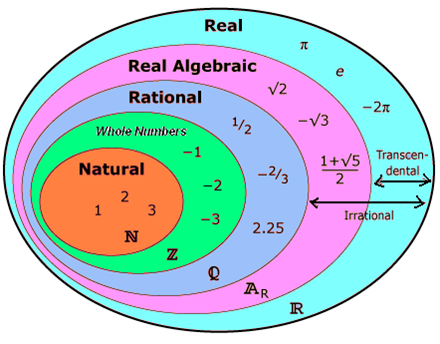
Decide if the following sentences are true or false:
a) All the Irrational Numbers are Real Numbers
True.
False.
b) All the Real Numbers are Rational Numbers
True.
False.
c) -3 is an irrational number
True.
False.
d) The Natural Numbers are Rational Numbers
True.
False.
Licensed under the Creative Commons Attribution Non-commercial Share Alike 3.0 License