sampling distribution of a proportion
When in a population we proceed to study a characteristic with only two possible values (success/failure), then the population follows a binomial distribution.
Each sample of the population has a percentage of individuals which has this characteristic. p is the proportion of success of this random variable in the population. The proportion of failure is q = 1 – p
Let all samples of size n in the population. Each sample has a proportion ![]() of individuals with this characteristic.
of individuals with this characteristic.
The distribution associated to the random variable that matches each sample with its proportion is called sampling distribution of a proportion.
As for big populations the binomial distribution approximates a normal one, the sampling distribution of a proportion follows a normal distribution, too
![]()
if n is greater enough, n ≥ 30, and np ≥ 5, nq ≥ 5
Because generally the proportions of the population are unknown, then we approximate it by the sample ones.
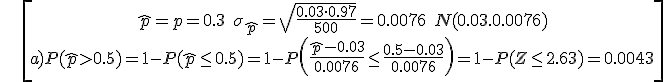
Licensed under the Creative Commons Attribution Non-commercial Share Alike 3.0 License