Limits calculation

Examples:

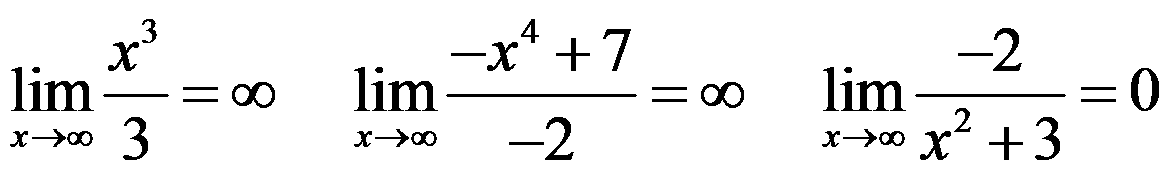
NOTE: remember that:

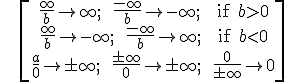
In infinite limits with polynomial functions, the limit is always ±∞ depending on the sign of the coefficient of the greatest degree term:

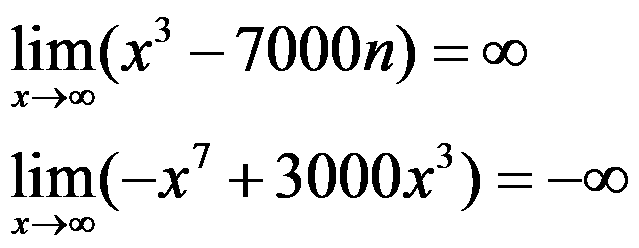
Undetermined forms of limits are the limits that are different depending on the situation. They are:

Infinite limits with rational functions always have an undetermined form of limit, which we have to solve by dividing by x to the highest power:

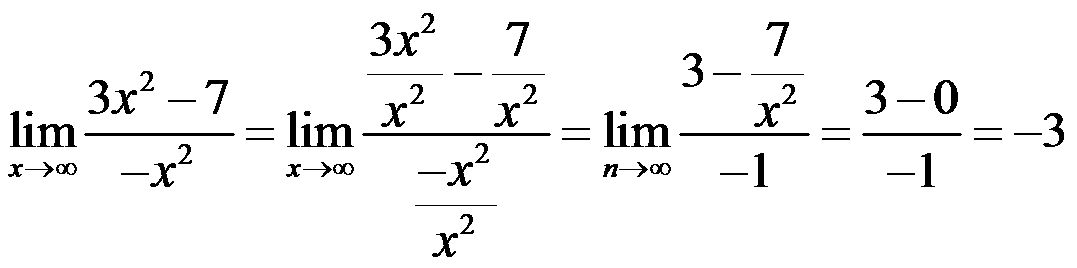
In the end:

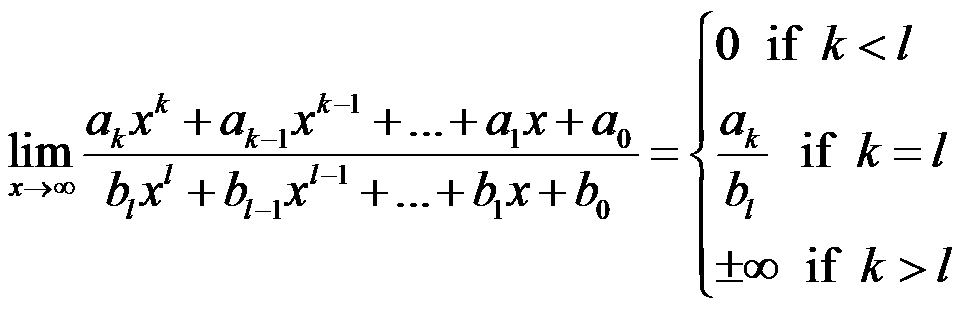
If we have irrational functions:

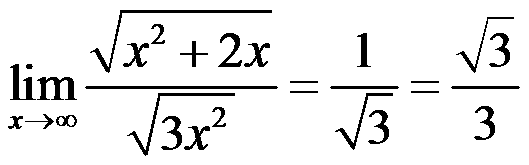
We can find an undetermined form of limit if we subtract square roots, then we have to use the conjugate to solve it:

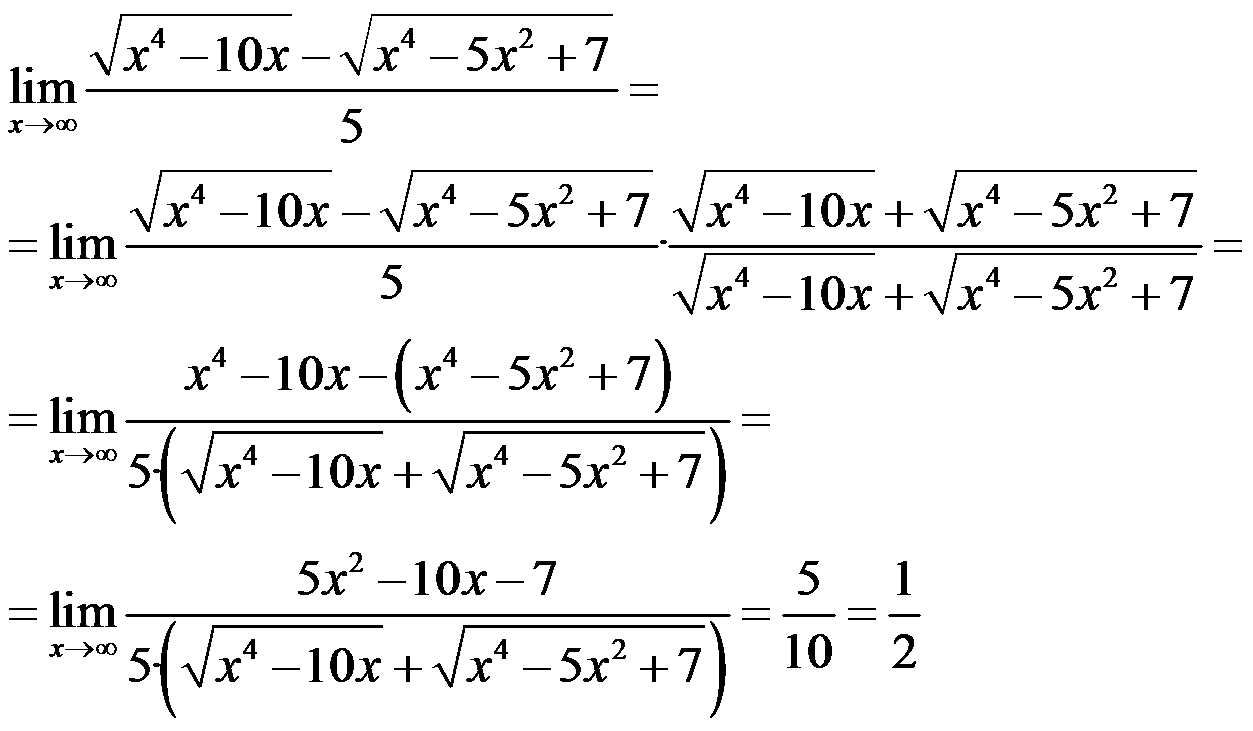
If a is -∞, then we change:

For example:

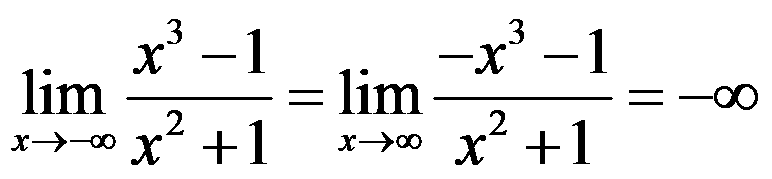
When a is not infinite, we can find other undetermined forms of limit:

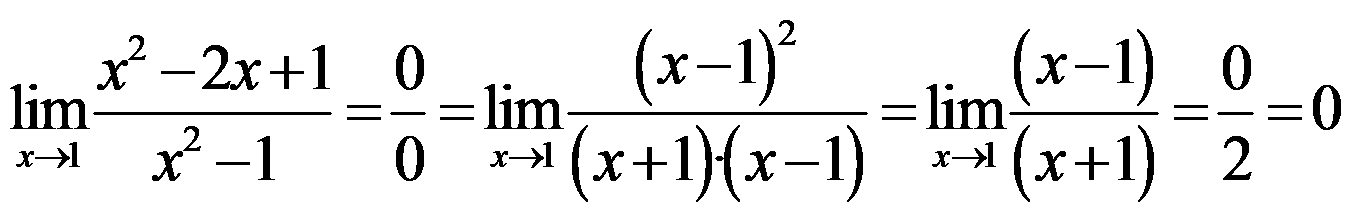
Other examples:

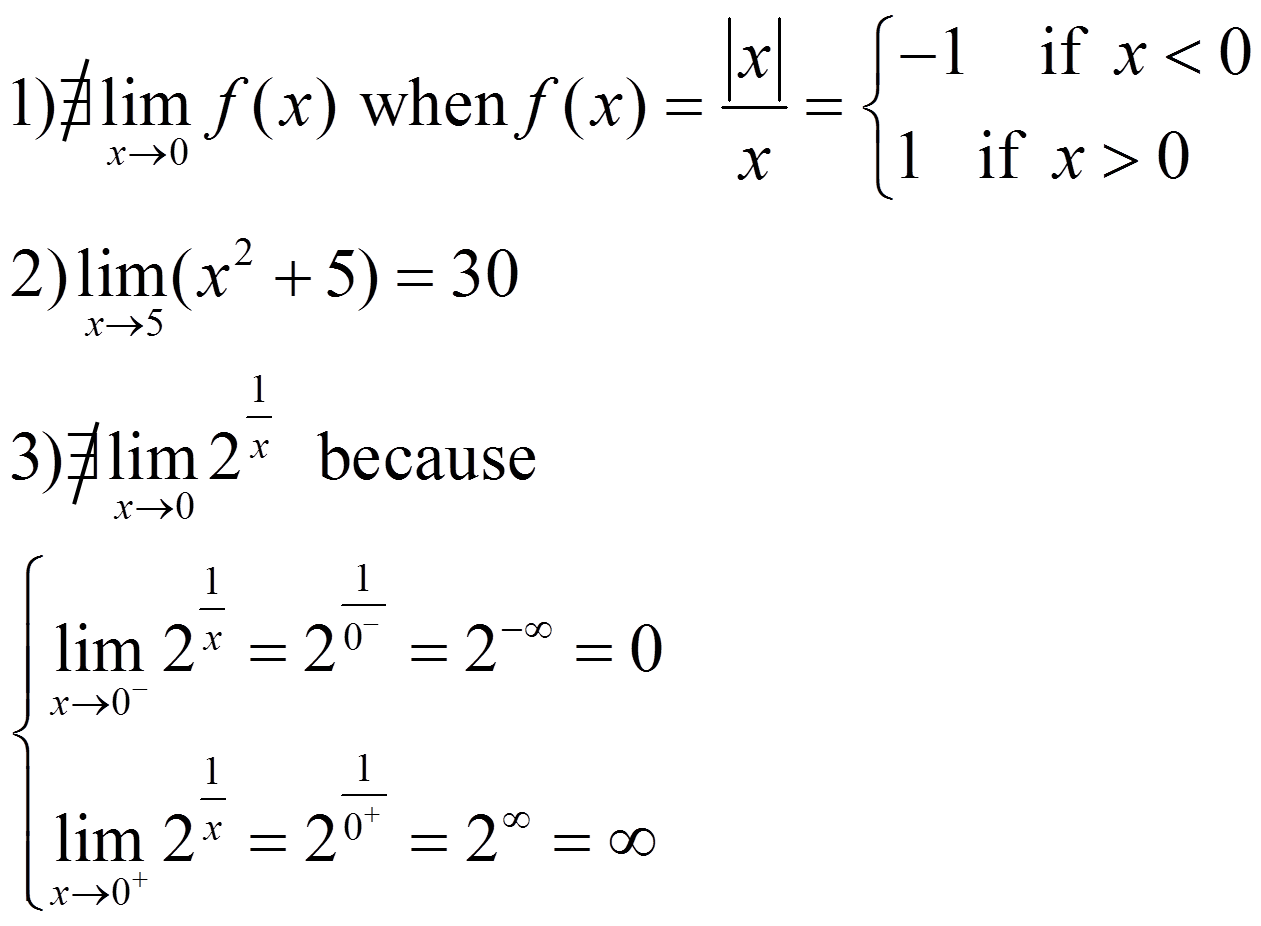
If the limit has powers, remember the property:

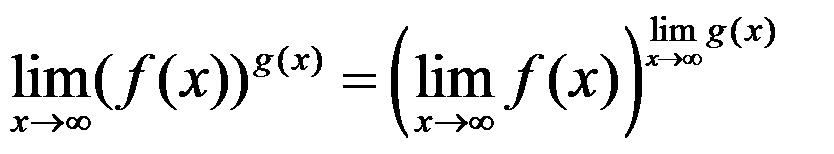
Examples:

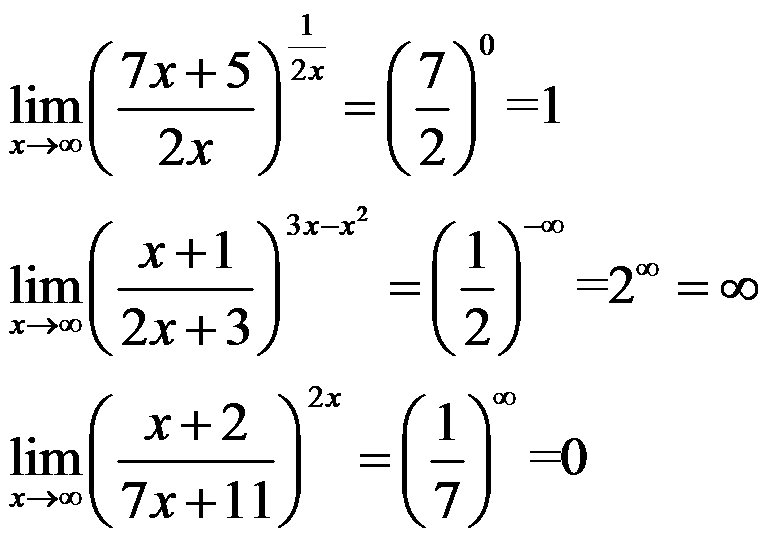
Number e is the limit:

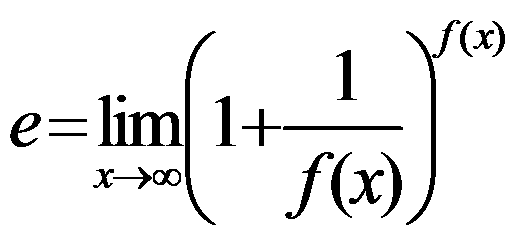
which is used in the undetermined form of limit 1^∞

Example:

Exercise: calculate:

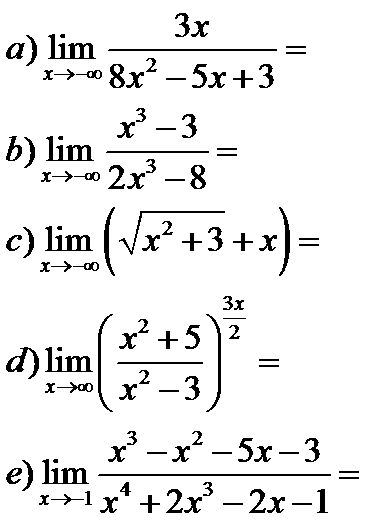
Solutions: a) 0; b) 1/2; c) 0; d) 1; e) -∞