Solving right triangles
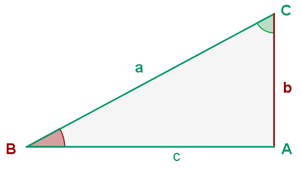
To solve a triangle is to find all its sides and all its angles. To do it in a right triangle, we have these formulas :

- Example 1:
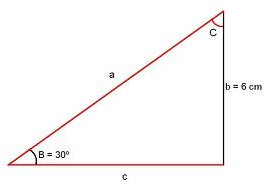 |
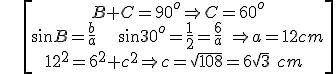 |
NOTE #1: To solve a triangle we need 3 data (they mustn’t be 3 angles)
NOTE #2: To find the angle when we have the trigonometric ratio, we use the sin-1, cos-1 and tan-1 keys in the calculator, pressing SHIFT or INV + sin, cos or tan.
- Example 2:
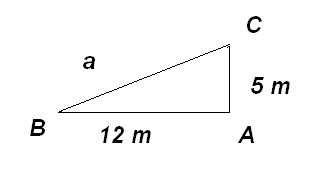 |
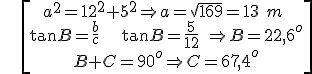 |
- Example 3: What is the angle of a 100% slope?
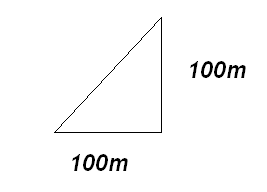 |
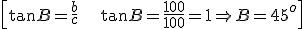 |
- Example 4: A flagpole stands in the middle of a flat, level field. Fifty feet away from its base, a surveyor measures the angle to the top of the flagpole as 48°. How tall is the flagpole?
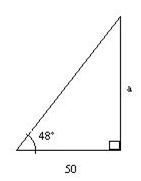 |
Let a denote the height of the flagpole. Then a / 50 = tan 480, so a = 50 tan 480 ≈ 55.5 ft |
- Example 5: Calculate the height of a tree knowing that, from a point on the ground, the top of the tree can be seen at an angle of 30o and from 10 m closer, the top can be seen at an angle of 60°.
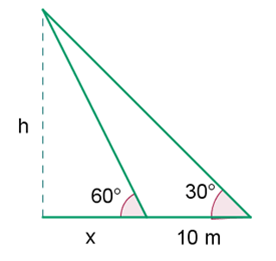 |
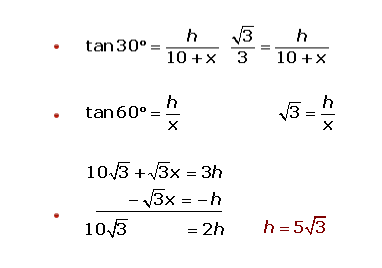 |
- Example 6: This photo was taken from a point about 500 m horizontally from the Opera House and we observe the waterline below the highest sail as having an angle of depression of 8°. How high above sea level is the highest sail of the Opera House?
 |
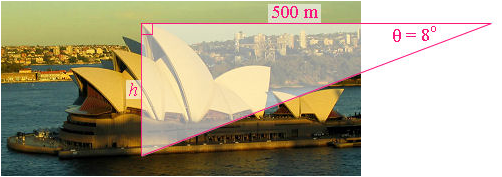 |
This is a simple tan ratio problem.
tan 8° = h/500 so h = 500 tan 8° = 70.27 m.
So the height of the tallest point is around 70 m.
[The actual height is 67.4 m.]
- Example 7: The length of the side of a regular octagon is 12 m. Find the radii of the inscribed and circumscribed circles.
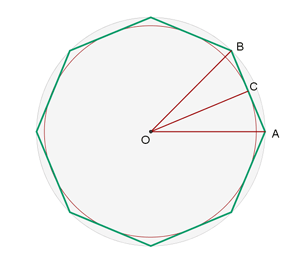 |
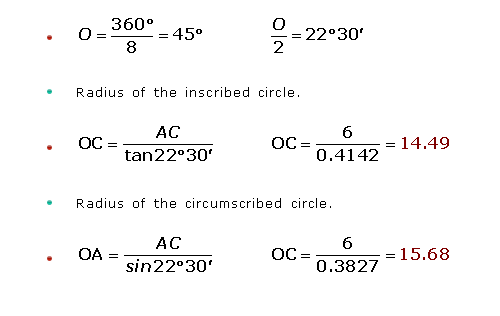 |
Exercises:
1.- The elevation angle of the Sun is 35o. Calculate the length of the shadow of a man of 1.75 m height.
2.- Calculate the height of a ladder of 4.5 m that rests against a wall, if the angle between the ladder and the floor is 67o.
Solutions: 1) 2.5 m; 2) 4.14 m
Licensed under the Creative Commons Attribution Non-commercial Share Alike 3.0 License