Continuity and derivability

THEOREM: If a function f is derivable in x = a, then f is continuous in x = a, too.

demonstration: we have to check that

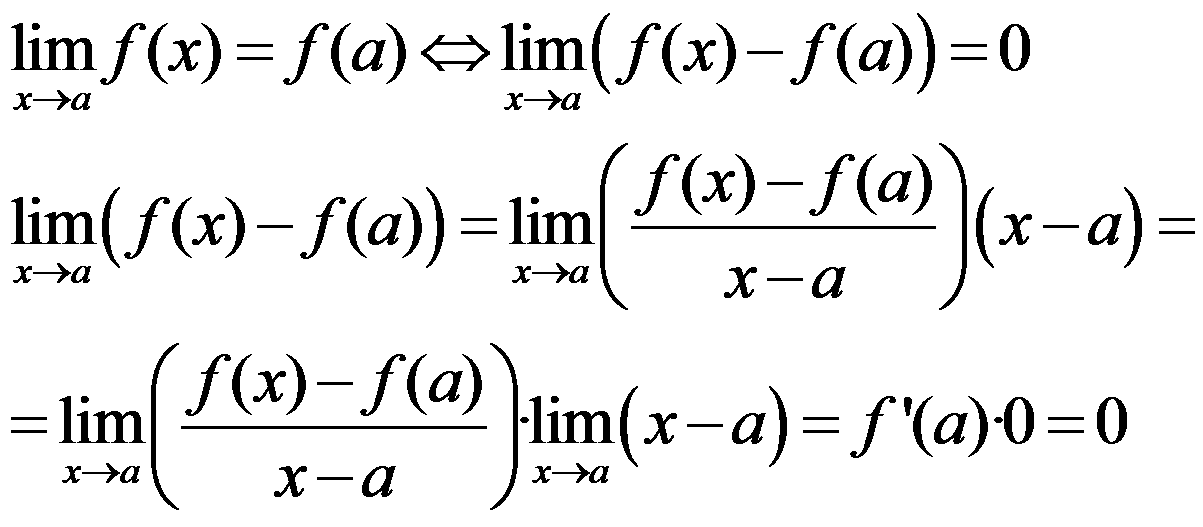
NOTE: Not all continuous functions in x = a are derivable in x = a.

Example: f(x) = |x| in x = 0

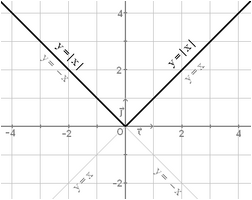
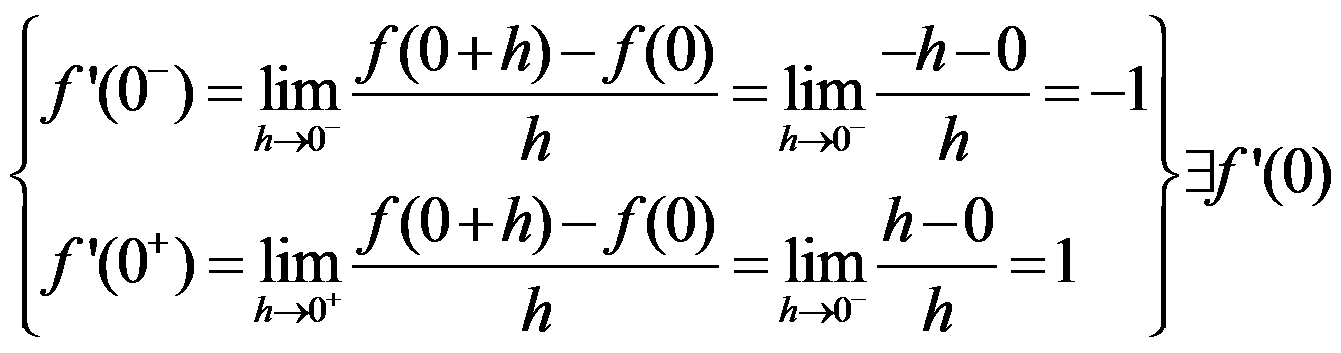
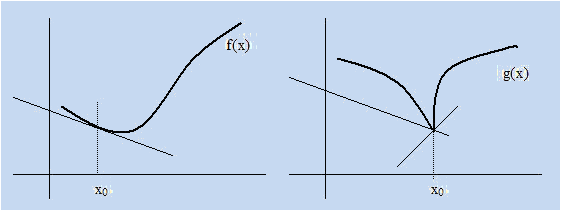
As you can see, derivability implies soft curves and non derivability implies peaks.

We define the derivative function as:

We only have to study the derivability of a function at the points which the function is continuous.

Example:

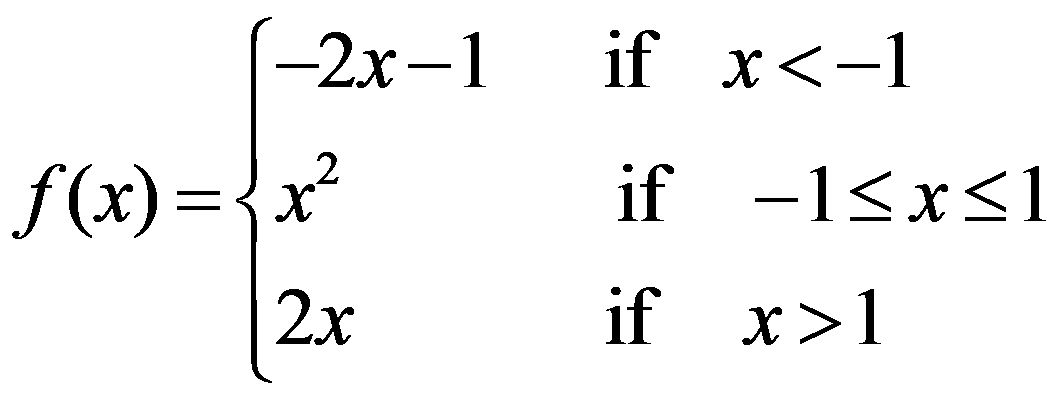
f is derivable in R-{1}

Exercises:

1) Find the derivative function of:

2) Find the abscissa in which the slope of the tangent line to the graph of f(x) = x² + 1 is 6.

Solutions: 1) f'(x) = -2/x²; b) f'(x) = 2x; 2) x = 3