DefiniteIntegralApplications
calculation of areas
- If f is positive:
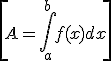
- If f is negative:
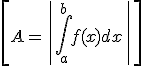
- If f is positive and negative, we have to find out its zeros in the interval: a1, a2, … ,ak / f(ai) = 0 and then:
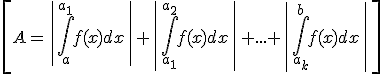
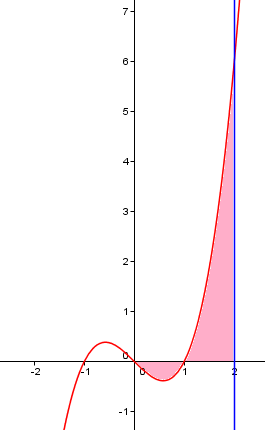
Example: calculate the area among the curve y = x3 – x, the abscissa axis, and the straight lines x = 0 and x = 2
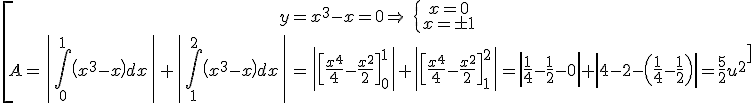
The area between the graphs of two functions, f and g, in [a,b]:
–If f ≤ g or f ≥ g in the interval
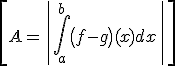
–Otherwise, we find out a1, a2, … ,ak in [a,b] / f(ai) = g(ai) and then:
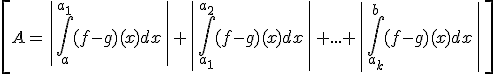
Example 1: calculate the area between the graphs of the functions: f(x) = x2, g(x) = -2x2 + 3

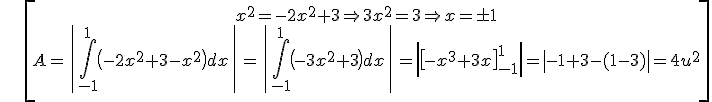
Example 2: calculate the area between the graphs of the functions: f(x) = x3, g(x) = 4x

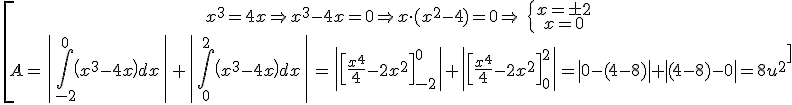
Exercises:
1.- Calculate the area among the graph of the function f(x) = x2 and the straight lines x = 1 and x = 3
2.- (PAEG- June 2012) Calculate the area enclosed by the parabola f(x) = x2 - 1 and the straight line g(x) = 5 - x
3.- (PAEG- September 2012) Calculate the area enclosed by the graphs of the functions f(x) = x3 - 3x2 + 2x + 1 and g(x) = 1
Solutions:
1.
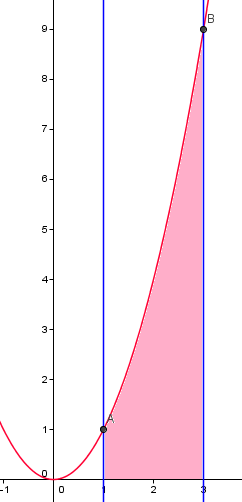 A = 26/3 u2
A = 26/3 u22.
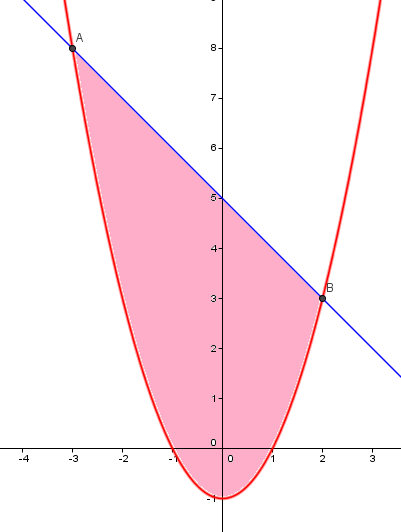 A = 125/6 u2
A = 125/6 u23.
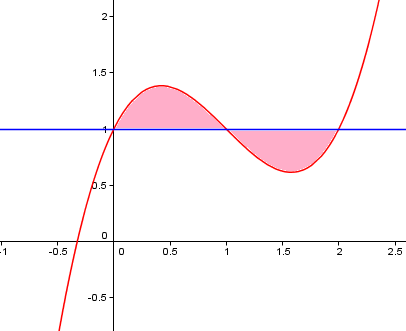 A = 1/2 u2
A = 1/2 u2
Licensed under the Creative Commons Attribution Non-commercial Share Alike 3.0 License