Approximations and errors

Frequently, we use approximate numbers because it isn’t necessary or convenient to give an exact quantity that we know, or maybe we cannot measure it exactly.

An estimate of a real number is another real number that is still close enough to be useful. An approximation of n-order of units is an approximation of the number in which we remove the digits of units of greater order. We can use the lower approximation or the higher one.

For example: the approximations of e = 2.718.. to the nearest hundredth are: 2,71 (lower) and 2,72 (higher)

The rounding of a number is the closest approximation to that number. Remember that in order to round a number to a particular order of units, we remove all the digits on the right of this order and, if the first substituted digit is greater than or equal to 5, we round up the previous digit too.
 
When we give an approximate number, we are making an error, which is the absolute value of the subtraction between the exact number and the approximate one. This is called absolute error.
 
For example, if we use 3.14 to approximate π, then: AE = |π – 3.14| = 0.001592…<0.01
 
In this example, we don’t know the exact error but we can control it. We can say the error is less than a hundredth.
 
To compare errors, we use the relative error:
 
 



 

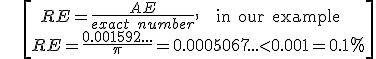
Exercise: Calculate the errors when we approximate 1/3 by 0.3 or e by 2.7

 

 

 

 

 

Solutions: a) AE = 1/30 = 0.0333...< 0.1; RE = 1/10 = 0.1 = 10%; b) AE = 0.018...< 0.1; RE = 0.00672...< 0.01 = 1%

Obra colocada bajo licencia Creative Commons Attribution Non-commercial Share Alike 3.0 License