Problems with conditions

Example 1: Calculate the value of a and b to make the function:

f(x) = ax3 + bx

have a relative minimum in (1,2)

 

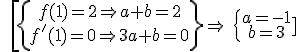
Example 2:     (PAEG - Reserve 2 - 2008) Determine the value of a,b€R, so that the function: f(x) =  asinx +  bcosx  passes through point (π/4,√2) and the slope of the tangent line at the point of abscissa x =π/2 is 5. Calculate the derivative of order 2008 of this function.
 
 
 
 
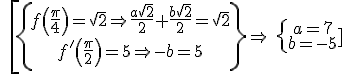
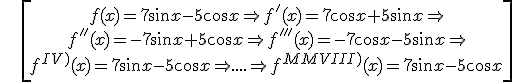
Exercise: Determine a,b,c to make the function f(x) = x3 + ax2 + bx + c have a maximum in  x=−4, a minimum in x=0 and it passes through the point (1,1).
 
 
 
 
 
 
Solution: a = 6, b = 0, c = -6

Licensed under the Creative Commons Attribution Non-commercial Share Alike 3.0 License