ellipse
The ellipse is the geometric place of all points in a plane whose distances to two fixed points, called foci, always add up to the same constant 2a.
d(P,F) + d(P,F’) = 2a
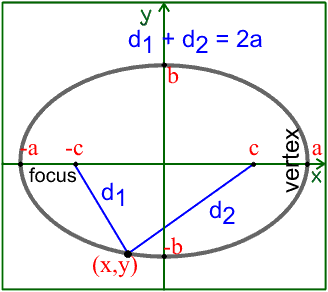
If C(c1,c2) is the center:
![]()
You can see that a2 = b2 + c2
The eccentricity of an ellipse is the quotient: e = c/a. You can check that 0 < e < 1, and if c is closer to 0, the ellipse looks like a circumference.
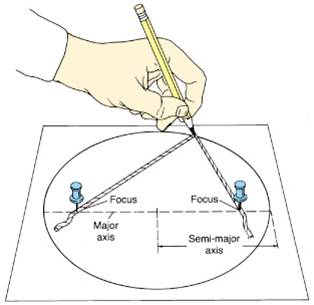
You can draw an ellipse as follows:
You can see ellipses:
- In the trajectory of Earth in its revolution movement around the Sun.
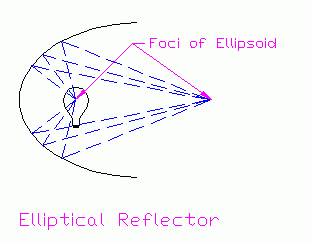
- You can check that a line from a focus to the ellipse is reflected to the other focus. This property is used in elliptical mirrors or in Medicine, to break kidney stones through sonic waves.
Exercise: Calculate the equation and the eccentricity of the ellipse with center (3,-2) and whose axis measure 12 and 18.
Solution:
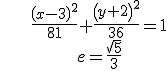
Licensed under the Creative Commons Attribution Non-commercial Share Alike 3.0 License