Remarkable points and straight lines in a triangle

The perpendicular bisectors in a triangle are the perpendicular bisectors of its sides. The three perpendicular bisectors of a triangle converge at a point called circumcenter, the center of the triangle’s circumcircle.

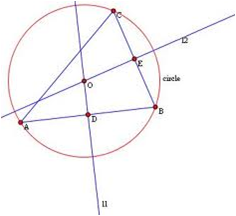
An angle bisector is the geometric place of all points which are equidistant to its sides. It is a line through a vertex that cuts its angle in half, too.

For example, if the sides of a triangle are r: 3x+ 4y = 0 and s:8x – 6y – 3 = 0, then

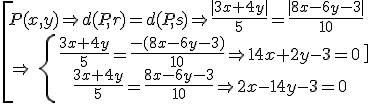
The three angle bisectors of a triangle converge at a point called incenter, the center of the triangle’s incircle.

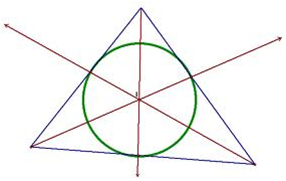
An altitude is a line through a vertex of a triangle and perpendicular to the opposite side. The three altitudes of a triangle converge at a point called orthocenter.

A median is a line that connects the vertex of a triangle to the midpoint of the opposite side. The three medians of a triangle converge at a point called centroid or geometric barycenter or center of mass.

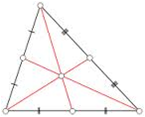
You can check that the coordinates of the center of mass of a triangle whose vertices are A(a1,a2), B(b1,b2) and C(c1,c2), are:

More of Euler line

Exercises:

1.- Find out the angle bisector of the angle that form the straight lines 3x - 4y + 10 = 0 and 5x - 12y -2 = 0

2.- Find the equation of the Euler line of the triangle that the points (-5,2), (4,7) and (6,-3) form.

Solutions: 1) x - 8y + 10 = 0, 8x + y +15 = 0; 2) 111 x - 55y - 75 = 0