hyperbola
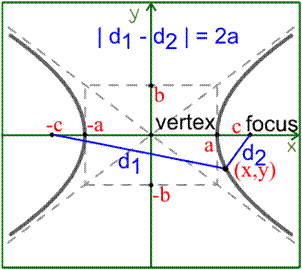
The hyperbola is the geometric place of all points in a plane whose difference between the distances to two fixed points, called foci, is the same constant 2a. It has two branches.
|d(P,F) - d(P,F’)| = 2a
If C(c1,c2) is the center:
![]()
You can see that a2 + b2 = c2 . Its asymptotes are: y = ±bx/a
The eccentricity of a hyperbola is the quotient: e = c/a. You can check that e > 1.
You can see hyperbolas:
- In hyperboloids and hyperbolic paraboloids, that are used in Architecture to build roofs or chimneys in nuclear power stations, for example.
- The hyperbolas are used in navigation in the LORAN (LOng RAnge Navigation) system. With this system, a boat receives signals from two knowing stations, and by measuring the difference of times, it is placed in the hyperbola corresponding to these foci. With another pair of stations, we obtain the position as the intersection of the two hyperbolas.
Exercise: Find the eccentricity and the equations of the asymptotes of the hyperbola:
![]()
Solutions:
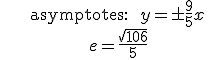
Licensed under the Creative Commons Attribution Non-commercial Share Alike 3.0 License