Examples

Example 1: Find the plane π and a straight line s that pass through the point (5,5,1) and are perpendicular to r:

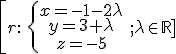
All straight lines in π are perpendicular to r, for example:

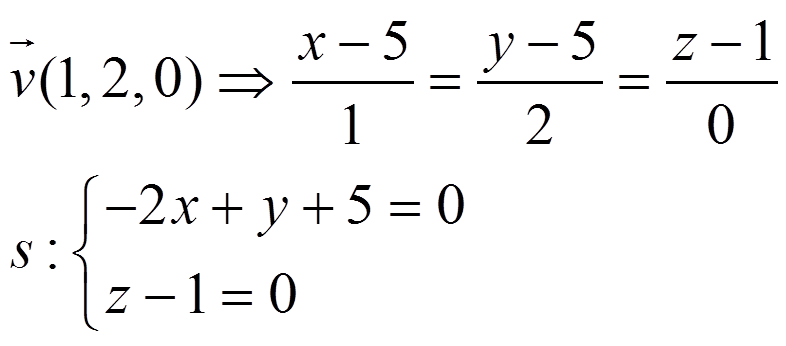
Example 2: Find the plane π that contains r and s:
 
 
 
 
 

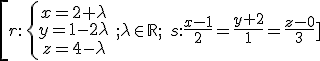
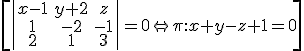
Example 3: Study, depending on a, the relative position of these lines:

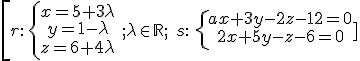
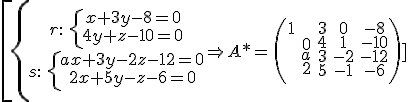
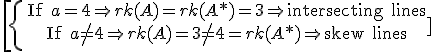
Example 4: Find the straight line t1 that passes through the point P(1,0,-1) and intersects r and s:
 

Find also the equation of another line, t2, perpendicular to both of them.

To obtain t1 we find the equation of two planes: one with r and P and another with s and P:

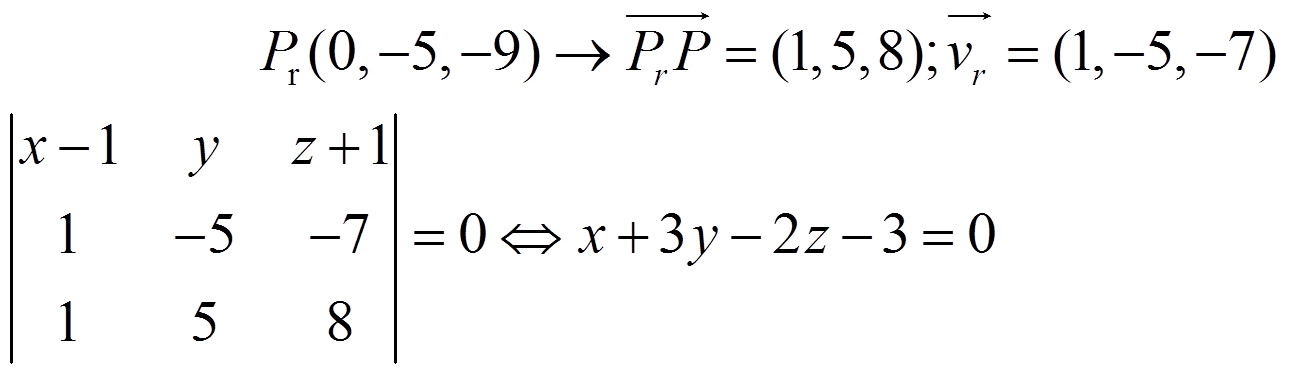
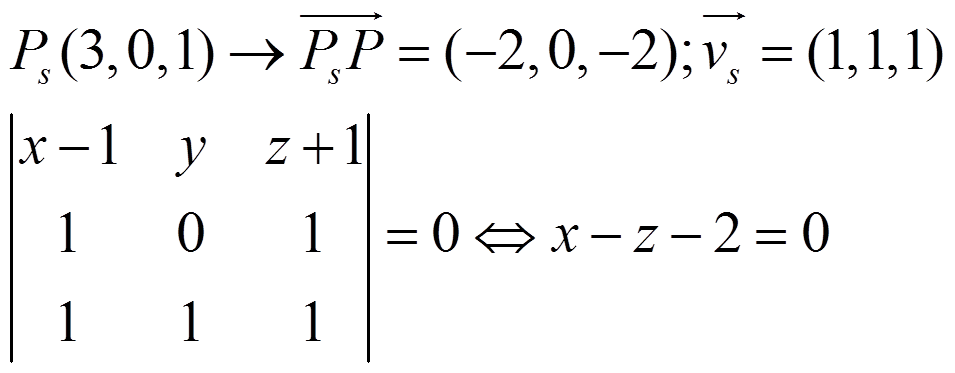
To obtain t2, we find its direction vector as:

Then, we find the equation of two planes: one with r and w and another with s and w:
 
 
 
 
Example 5: Find the coordinates of the symmetric point of:
 
- the point P(-1,1,-1) from the line:
 

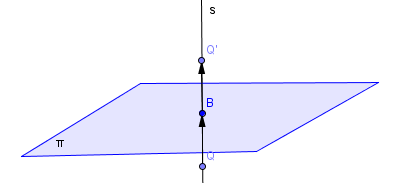
- the point Q(2,2,-3) from the plane π: 3x – y + 2z – 8 = 0
 
For the first one, we must find the point A. To do that, we find the plane π’ perpendicular to r that passes through P:
 
 
 
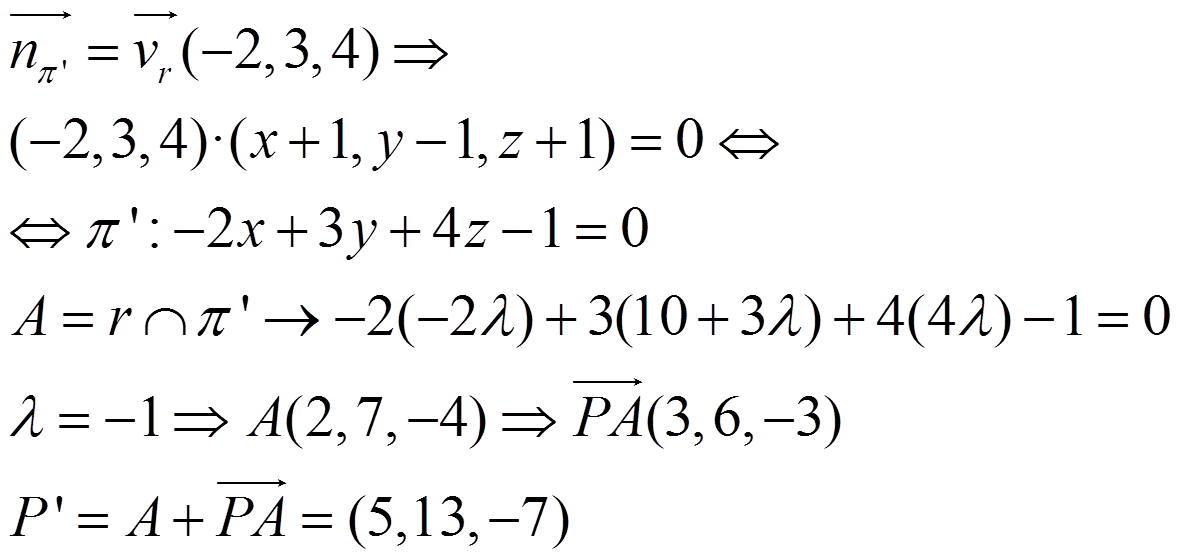
For the second one, we must find the point B. To do that, we find the line s perpendicular to π that passes through Q:

 Exercises:
 
1.- (PAEG- September 2013)
 
a) Let the points P(4, 2, 3) and Q(2, 0,-5). Find the equation of the plane π that makes Q the symmetric point of P from the plane π.

b) Calculate the value of λ€R so that the plane determined by the points P, Q, and R(λ,1,0) passes through the origin.


2.- (PAEG- Reserve 1 - 2013) Let the planes: π: ax + 2y + z - 4 = 0 (a€R) and π´: 2x - 4y - 2z - b = 0 (b€R).

a) Find, in a reasoned way, the values of a and b that make π and π' coincident planes.

b) Find, in a reasoned way, the values of a and b that make π and π' parallel and non-coincident planes.

c) Find, in a reasoned way, the values of a and b that make π and π' perpendicular planes.



Solutions: 1) a) x + y + 4z = 0; b) λ = 13/5; 2) a = -1, b = -8; b) a = -1, b ≠ -8; c) a = 5

Obra colocada bajo licencia Creative Commons Attribution Non-commercial Share Alike 3.0 License