Limits of functions
Functions
A function f, is a relation between two sets with the property that each element in the first set is related to exactly one element in the second set.
A real-valued or real function is a function whose sets are the Real Numbers:
![]()
The domain of a function is the subset of the initial set of the elements that have an image.
The range or image is the subset of the final set (or codomain) of the elements that are images of an element of the domain.
Example 1:
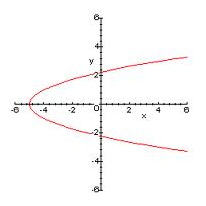 it is not a function
it is not a functionExample 2:
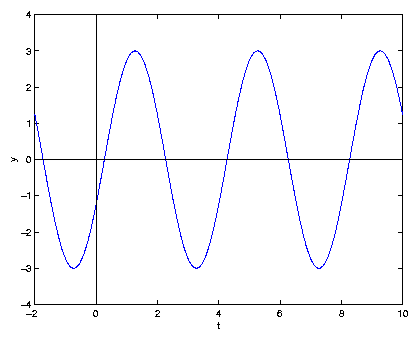 Domain = R Range = [-3,3]
Domain = R Range = [-3,3]Example 3: Whole number portion function
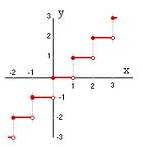
Domain = R Range = Z
Exercise: Decide if the following relations are functions or not, and if they are, find their domain and range:
a)

b)

c)

Solutions:
a) it's a function, dom = [-1,2]U[3,5)U(5,8], range = [0,5]
b) it's a function, dom = range = R
c) it is not a function
Licensed under the Creative Commons Attribution Non-commercial Share Alike 3.0 License