If we want to estimate the weight of a friend and say «80 kg», we are making an estimate that says nothing about how certain this is so.
On the other hand, if we say: "I am sure that you weigh between 75 and 85 kg", or "I am almost certain that your weight is between 78 and 87 kg», we convey that there is a certain degree of certainty about the weight being between those values.
Logically the greater the interval, the greater the degree of confidence that we can have, although the error with which we estimate is also higher.
Let’s consider a long series of samples of size n from a population of mean μ and σ standard deviation.
We know that the sampling distribution of means follows a normal N(μ,σ/√n).
If
we are stating that the probability that, once extracted a sample of size n randomly, we are 99% confident that the mean of this sample is in the interval
That is, if you have a large number of samples of size n in the same conditions, in 99% cases of the interval similar to the given one, it would include the true value of the μ parameter and 1% cases it would not.
These intervals are called confidence intervals for a confidence level, corresponding to a critical value.
The confidence level, 1-α, involves that (1-α)·100% of the confidence intervals contain the population parameter that we are estimating. To each confidence level, CL, belongs a critical value zα/2 related to the normal distribution N(0,1) and which verifies:
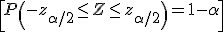
The extremes of the interval are called confidence limits.
α is called significance level.
To build the confidence interval in which there is a population parameter with a level of confidence CL we have to follow the following steps:
–Determine the sample estimate
–Determine the standard deviation corresponding to the estimate
–Determine the critical value zα/2 corresponding to the confidence level CL
–Then, desired confidence interval is (estimate - zα/2·SD, estimate + zα/2·SD)
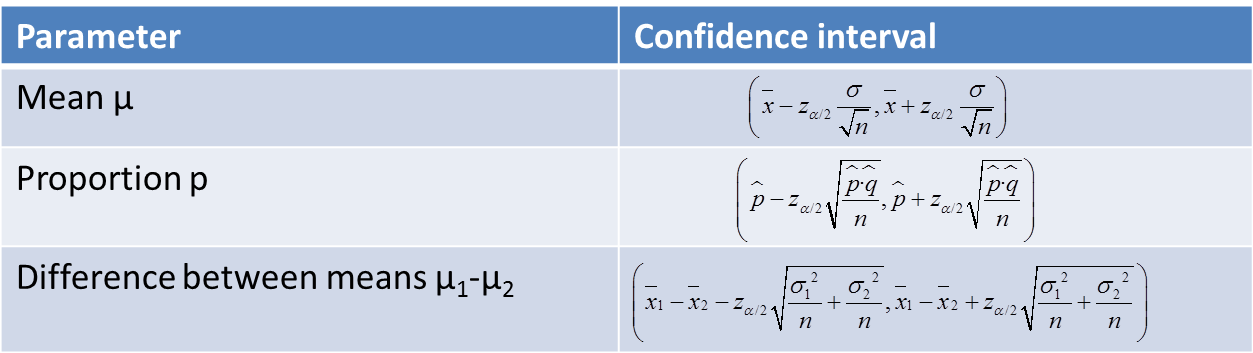
The confidence intervals, depending on the population parameter that we estimate, are:
To determine the critical value if we set, for example, a confidence level CL = 99%, it has to verify:
Then
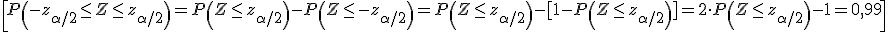
and, if we work out:
By using the normal table, we deduce that zα/2=2.58
Generally, we can say that the critical value zα/2 corresponding to a confidence level CL, is calculated by using the expression
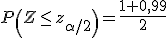
and, then, using the normal table
Example 1: A random sample of 100 students who took the PAEG exam revealed that the average age is 18.1 years. Find a confidence interval of 90% for the mean age of all students who attended the exam, knowing that the standard deviation of the population is 0.4.
Sample estimator is sample mean
and
The confidence interval in which the mean μ of all students is located is:
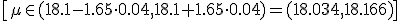
Example 2: To estimate the proportion of students from a University that is in favour of the social reintegration of offenders, 500 students were randomly interviewed. 58 were in favour. Calculate the confidence interval, with significance level of 5%, in which the University population that is in favour must be found.
As n=500, then we can approximate p and q with the proportions of the sample

Then, the interval is
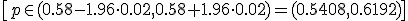
Example 3: You want to provide a sports hall with a good lighting system. For this aim, two samples of lamps from two different factories are analysed. Examined the first sample of 100 lamps, they show a mean life of 1 500 hours with a standard deviation of 150 hours. Sample of 130 lights from a second manufacturer offers a mean life of 1380 hours, with a standard deviation of 70 hours. Find the confidence limits for the difference with a confidence level of 99%. What will the chosen lamp be?
The means and SD of the population are unknown. n≥100 in both samples, then we can approximate the SD of the population by the sample ones. Then

And the confidence interval is
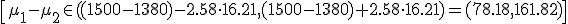
As the void difference between means is not in the interval, there is a positive difference between both lamps. Therefore we should choose the first one.
![]()
![]()
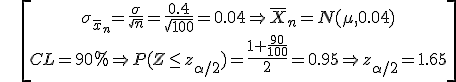
![]()
![]()
![]()