hypothesis test for difference between means

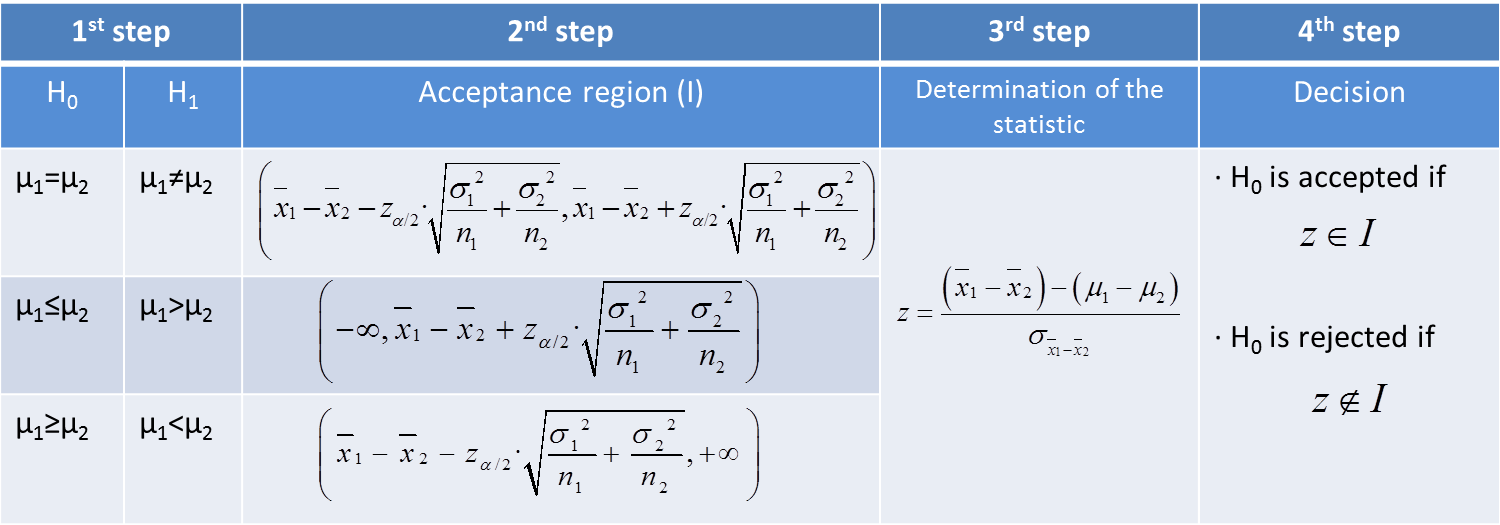
In this case, as in the mean case, it all begins with an “a priori” assumption that the value of both means is equal, μ1 = μ2. Then we use

which are calculated by obtaining a random sample from both populations in order to decide if the assumption that μ1 = μ2 is likely. We will follow these steps:

Example: We studied two samples (A and B) of Andalusian citizens of 80 members each, to know about their nationalistic sentiment. On a scale of 1 to 10, the first group averaged 7.2 with standard deviation of 3.1, while the second group averaged 8.1 with a standard deviation of 4.2. Our research hypothesis is that group B has a greater nationalist sentiment than collective A. Check the hypothesis for a significance level of 0.01.
 
Step 1: H0: μ1 ≥ μ2; H1: μ1 < μ2
 
Step 2: This is a one-tailed test with α = 0.01, then the critical value zα = -2.33
 
Step 3: n is great enough, so we can apply the distribution N(0, 1)
 

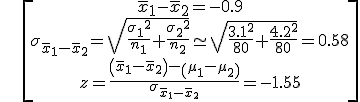
Step 4:
We reject the alternative hypothesis; therefore, there are no significant differences between the level of nationalist sentiment for both groups

Licensed under the Creative Commons Attribution Non-commercial Share Alike 3.0 License