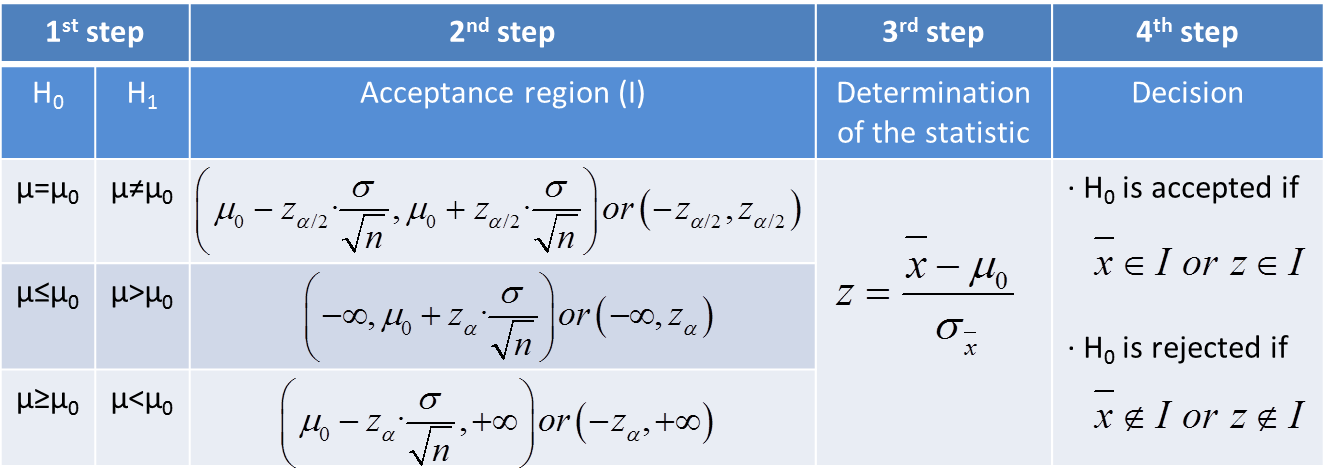
Inferential Statistics
hypothesis test for mean
In these situations we must begin by an “a priori” assumption of the mean value of the population, μ. Then we use , the sample mean, which is calculated by obtaining a random sample from the population, to decide whether this assumption about μ is likely. We must follow the steps outlined for hypothesis testing:
Example: A survey of 64 professionals working for an institution revealed that the mean time of employment in that field was 5 years, with a standard deviation of 4. Considering a significance level of 0.05, do these data support the fact that the mean time of employment of these professionals is below 6 years? We assume that professional population is normally distributed.
Step 1: H0: μ ≥ 6; H1: μ < 6. The sample data make us infer that the mean time of employment is less than 6 years, which marks the direction of the test
Step 2: This is a one-tailed test with α = 0.05, then the critical value zα = -1.645, which separates the rejection and non-rejection regions. The acceptance interval is: I = (5.1775,+∞)
Step 3: The sampling distribution of reference is set to normal. Then, we calculate the value of z corresponding to the distribution N(0,1)
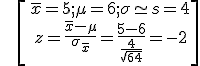
Step 4:

Then, the mean time of employment is lower than 6 years
Licensed under the Creative Commons Attribution Non-commercial Share Alike 3.0 License