Inferential Statistics
Statistical hypothesis test
We call statistical hypothesis tests to the procedures that allow you to decide if a hypothesis is accepted or rejected, or determine if observed samples differ significantly from the expected results.
The process to be followed in the statistical hypothesis test has these four steps:
–Step 1: Formulate the null and alternative hypotheses. Both hypotheses are mutually exclusive. The null hypothesis, H0, is the one you have to contrast and it is usually formulated with the idea of being rejected. Depending on the formulating of the alternative hypothesis, H1, the proof of the hypothesis will be bilateral or unilateral.

–Step 2: Determine the rejection and non-rejection (acceptance) region for a determined significance level α. That level is the probability of rejecting the null hypothesis if it is true. α let us limit the rejecting and non-rejecting regions.
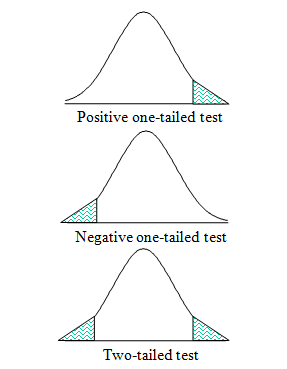
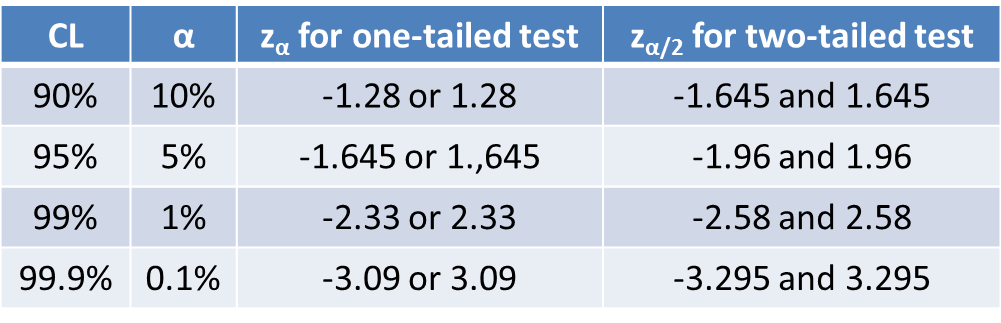
–Step 3: Choose the suitable statistic. The statistics that we are going to use are the sample mean, the sample proportion and the difference between sample means. All of them follow a normal distribution. Once we have determined the sample distribution, we define the rejecting region for the null hypothesis.
–Step 4: Make a decision and interpret it. According to the results, we will accept the null hypothesis, with a significance level of α, if this statistic is within the non-rejection region and, otherwise, it will be rejected.
Licensed under the Creative Commons Attribution Non-commercial Share Alike 3.0 License