Inferential Statistics
Size of the samples. Maximum permissible error
When we study the confidence interval, we see that its amplitude depends on these terms:
![]()
That is, the greater the size of the sample is the more reliable the interval is but, conversely, the more expensive the study is.
Then the target is to find a good size which, with a reasonable expense, can give us reliable results and limit the maximum permissible error:
![]()
Then, the maximum permissible error verifies:
–The more confidence level, the more zα/2 and therefore, the greater error
–The bigger size of the sample, the lower error
We can obtain the size of the sample through expressions of the error:
–To estimate the means:
–To estimate the proportions:
Example 1: It is known youngsters’ mean of time devoted to leisure follows a normal distribution of mean 400 minutes and standard deviation of 63 minutes. Find what is the minimum sample size of youths that guarantees, with a probability of 0.95, that the mean leisure time is between 382 and 418 minutes.
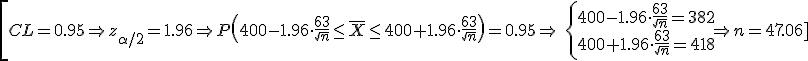
Then, the size of the sample must be 48
Example 2: We want to know the number of people over the legal age that would be necessary to include in a national sample with an absolute error of E = 0.04 and a confidence level of the 99.73%. Last census has a value of P = 0.45.
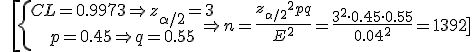
Then, the size of the sample must be 1392
Licensed under the Creative Commons Attribution Non-commercial Share Alike 3.0 License