5. periodic functions

A function f is said to be periodic with a period T if, for some nonzero constant T, we have:

f(x + T) = f(x)  for all values of x.

If there exists a least positive constant T with this property, it is called the prime period. A function with period T will repeat on intervals of length T, and these intervals are sometimes also referred to as periods.

Example: f(x) = sin x, is a periodic function and its prime period is T = 2π

sin (x + 2π) = sin x

 

 

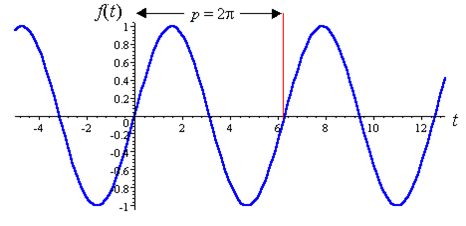
Exercise: decide whether these functions are periodic or not and if they are, find their prime period:

a)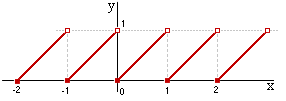 

b)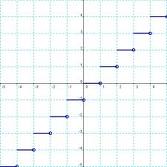 

c)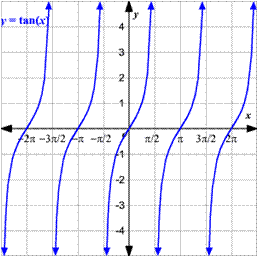 

 

 

 

Solutions: a) periodic, T = 1; b) it is not a periodic function; c) periodic, T = π