8. extrema, increasing and decreasing

A function is said to be increasing in an interval if, for all x₁ and x₂ in the interval such that x₁ < x₂, then f(x₁) < f(x₂).

A function is said to be decreasing in an interval if, for all x₁ and x₂ in the interval such that x₁ < x₂, then f(x₁) > f(x₂).

The maximum and minimum of a function, known collectively as extrema, are the largest and smallest value that the function takes at a point either within a given neighborhood (local or relative extremum) or on the function domain in its entirety (global or absolute extremum).

Examples:

 

 

 

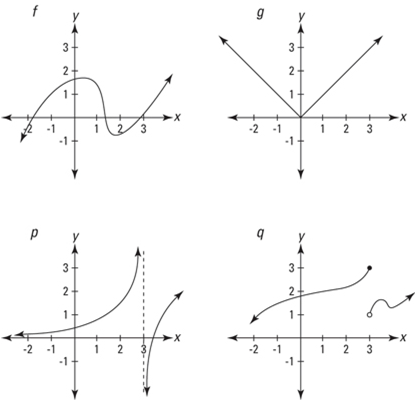
Exercise: study the extrema, increasing and decreasing of these functions:

a)

b)

 

 

 

 

Solutions: 

function	abs max	abs min	rel max	rel min	increasing	decreasing
a)	Φ	0	Φ	0	(0,2)	(-∞,0)U(2,6)
b)	2	-6, 6	-3.5 , 2	-1.5	(-6,-3.5)U(-1.5,2)	(-3.5,-1.5)U(2,6)